溫馨提示×
您好,登錄后才能下訂單哦!
點擊 登錄注冊 即表示同意《億速云用戶服務條款》
您好,登錄后才能下訂單哦!
二叉查找樹(Binary Search Tree),也稱有序二叉樹(ordered binary tree),排序二叉樹(sorted binary tree),是指一顆空樹或者具有下列性質的二叉樹:
(1)每個節點都有一個作為搜索依據的關鍵碼(key),所有的節點的關鍵碼互不相同。
(2)左子樹上所有的關鍵碼(key)都小于根節點點的關鍵碼(key)。
(3)右子樹上所有的關鍵碼(key)都大于根節點的關鍵碼(key)。
(4)左右子樹都是二叉搜索樹。
代碼實現如下:
#include<iostream>
using namespace std;
template<class K,class V>
struct BSTreeNode{
BSTreeNode<K, V>* _left;
BSTreeNode<K, V>* _right;
K _key;
V _value;
BSTreeNode(const K& key,const V& value)
:_key(key)
, _value(value)
, _left(NULL)
, _right(NULL)
{}
};
template<class K,class V>
class BSTree{
typedef BSTreeNode<K, V> Node;
public:
BSTree()
:_root(NULL)
{}
//非遞歸
bool Insert(const K& key, const V& value)
{
if (_root == NULL)
{
_root = new Node(key,value);
return true;
}
Node* parent = _root;
Node* cur = _root;
while (cur)
{
if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
else if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
else
{
return false;
}
}
if (parent->_key>key)
{
parent->_left = new Node(key,value);
}
else
{
parent->_right = new Node(key, value);
}
return true;
}
void InOrder()
{
_InOrder(_root);
cout << endl;
}
Node* Find(const K& key)
{
if (_root == NULL)
{
return NULL;
}
Node* cur = _root;
while (cur)
{
if (cur->_key > key)
{
cur = cur->_left;
}
else if (cur->_key < key)
{
cur = cur->_right;
}
else
{
return cur;
}
}
return NULL;
}
bool Remove(const K& key)
{
if (_root == NULL)
{
return false;
}
Node* parent = NULL;
Node* cur = _root;
while (cur)
{
if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
else if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
else
break;
}
if (cur == NULL)
return false;
Node* del;
//刪除節點的左為空
if (cur->_left == NULL)
{
del = cur;
if (parent == NULL)
{
_root = cur->_right;
}
else
{
if (parent->_left == cur)
{
parent->_left = cur->_right;
}
else
{
parent->_right = cur->_right;
}
}
delete del;
}
//刪除節點的右為空
else if (cur->_right == NULL)
{
del = cur;
if (parent == NULL)
{
_root = cur->_left;
}
else
{
if (parent->_left == cur)
{
parent->_left = cur->_left;
}
else
{
parent->_right = cur->_left;
}
}
delete del;
}
//刪除節點的左右都不為空
else
{ //找右樹的最左節點,也就是右邊最小的數
parent = cur;
Node* left = cur->_right;
while (left->_left)
{
parent = left;
left = left->_left;
}
del = left;
cur->_key = left->_key;
cur->_value = left->_value;
if (parent->_left == left)
{
parent->_left = left->_right;
}
else
{
parent->_right = left->_right;
}
delete del;
}
return true;
}
//遞歸
Node* FindR(const K& key)
{
return _FindR(_root,key);
}
bool InsertR(const K& key, const V& value)
{
return _InsertR(_root,key,value);
}
bool RemoveR(const K& key)
{
return _RemoveR(_root,key);
}
protected:
void _InOrder(Node* root)
{
if (root != NULL)
{
_InOrder(root->_left);
cout << root->_key << " ";
_InOrder(root->_right);
}
}
Node* _FindR(Node* root,const K& key)
{
if (root == NULL)
{
return NULL;
}
if (root->_key == key)
{
return root;
}
if (root->_key > key)
{
return _FindR(root->_left,key);
}
else
{
return _FindR(root->_right,key);
}
return NULL;
}
bool _InsertR(Node*& root, const K& key, const V& value)
{
if (root == NULL)
{
root = new Node(key,value);
return true;
}
if (root->_key > key)
{
return _InsertR(root->_left,key,value);
}
else
{
return _InsertR(root->_right,key,value);
}
return false;
}
bool _RemoveR(Node*& root, const K& key)
{
if (root == NULL)
{
return false;
}
if (root->_key > key)
{
return _RemoveR(root->_left,key);
}
else if (root->_key < key)
{
return _RemoveR(root->_right,key);
}
else
{
//刪除的節點的左為空
if (root->_left == NULL)
{
root = root->_right;
}
//刪除節點的右為空
else if (root->_right == NULL)
{
root = root->_left;
}
else
{ //找右邊最左的節點(即右邊最小的節點)替換刪除的該節點(下面程序采用的)。
//或者找左邊最右的節點(即左邊最大的節點)替換刪除的該節點
Node* parent = root;
Node* left = root->_right;
while (left->_left)
{
parent = left;
left = left->_left;
}
root->_key = left->_key;
root->_value = left->_value;
if (parent->_left == left)
{
parent->_left = left->_right;
}
else
{
parent->_right = left->_right;
}
}
return true;
}
return false;
}
protected:
Node* _root;
};
#include "BSTree.h"
void Test1()
{
int arr[10] = { 0, 1, 3, 5, 4, 2, 7, 8, 6, 9};
BSTree<int, int> bst;
for (int i = 0; i < sizeof(arr) / sizeof(arr[0]); ++i)
{
bst.Insert(arr[i],i);
}
bst.InOrder();
BSTreeNode<int, int>* ret1=bst.Find(8);
if (ret1)
{
cout << ret1->_key << ":" << ret1->_value << endl;
}
else
cout << "沒有找到ret1" << endl;
BSTreeNode<int, int>* ret2=bst.Find(22);
if (ret2)
{
cout << ret2->_key << ":" << ret2->_value << endl;
}
else
cout << "沒有找到ret2" << endl;
bst.Remove(9);
bst.Remove(7);
bst.Remove(8);
bst.InOrder();
bst.Remove(0);
bst.Remove(1);
bst.Remove(2);
bst.Remove(3);
bst.Remove(4);
bst.Remove(5);
bst.Remove(6);
bst.Remove(7);
bst.Remove(8);
bst.Remove(9);
bst.InOrder();
}
void Test2()
{
int arr[10] = { 0, 1, 3, 5, 4, 2, 7, 8, 6, 9 };
BSTree<int, int> bst;
for (int i = 0; i < sizeof(arr) / sizeof(arr[0]); ++i)
{
bst.InsertR(arr[i], i);
}
bst.InOrder();
BSTreeNode<int, int>* ret1 = bst.Find(7);
if (ret1)
{
cout << ret1->_key << ":" << ret1->_value << endl;
}
else
cout << "沒有找到ret1" << endl;
BSTreeNode<int, int>* ret2 = bst.Find(12);
if (ret2)
{
cout << ret2->_key << ":" << ret2->_value << endl;
}
else
cout << "沒有找到ret2" << endl;
bst.RemoveR(8);
bst.RemoveR(7);
cout<<bst.RemoveR(9)<<endl;
bst.InOrder();
bst.RemoveR(0);
bst.RemoveR(1);
cout << bst.RemoveR(2) << endl;
bst.RemoveR(3);
bst.RemoveR(4);
bst.RemoveR(5);
bst.RemoveR(6);
bst.RemoveR(7);
cout << bst.RemoveR(8) << endl;
bst.RemoveR(9);
bst.InOrder();
}
int main()
{
Test1();
Test2();
return 0;
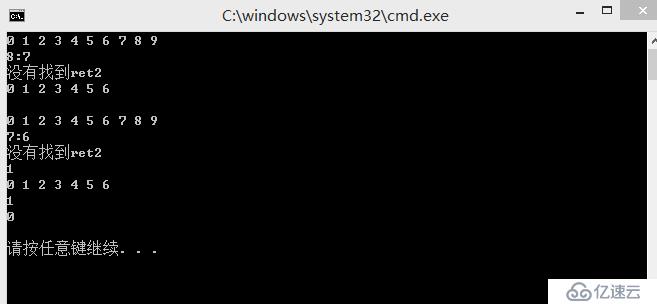
}運行結果:
免責聲明:本站發布的內容(圖片、視頻和文字)以原創、轉載和分享為主,文章觀點不代表本網站立場,如果涉及侵權請聯系站長郵箱:is@yisu.com進行舉報,并提供相關證據,一經查實,將立刻刪除涉嫌侵權內容。